5 way venn diagrams

I'm rather pleased with myself, though it's hardly deserved. I was working on 4-data set Venn diagrams about a month ago and told Steve that "this is as high as I think we can go. We won't be able to visualize any higher order data set overlaps", and then managed to prove myself wrong today.

Taking inspiration from another site on the web showing triangular based Venn diagrams for 5-data sets, I came up with a much cleaner representation:



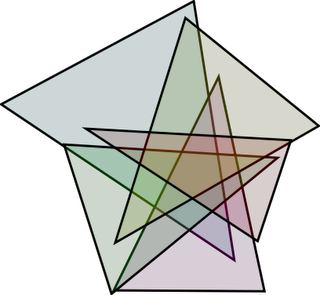
It's not like I invented a new branch of math or anything, but hey, that's pretty darn cool. The triangle sizes are roughly proportional with the size of the datasets, which I didn't even think i could approximate, and I even like the look of it. While I knew this wouldn't be possible with circles (I had played briefly with rhomboids and triangles before), I was stuck until I saw this picture, and then realized that there was a much cleaner way to assemble the triangles using parallel edges, and tada, we had a winner.

Obviously, the more generalized version using identical sized triangles can be done as well. In inkscape, it's relatively easy make a 3 sided polygon, copy it 4 times and then rotate each one by 72 degrees and layer them with some opacity. Aren't Venn diagrams fun?

I hear the chorus calling me a geek, so I'll move on to other topics.

I'm currently planning on resuming my blogging with more frequent updates. The last month was a good break, giving me a bit more time to catch up on other things (research), but I've saved up several topics, and I'm ready to get going again. I'll try for at least 3 a week for the next while. Wish me luck.